
Note: The output for \(R_1\) is the same as for Ex3.3, but inverted. Download our understanding cryptography even solutions eBooks for free and learn more about understanding cryptography even solutions. Public Key Infrastructure for OTP strong authentication. Show that S 1 ( x 1) S 1 ( x 2) S 1 ( x 1 x 2), where denotes bitwise XOR, for: x 1 000000 2, x 2. Display payment cards can even integrate an OTP generator for 2-factor authentication. In this problem we verify this property by computing the output of S1 for several pairs of inputs. Note that you can also try values x i which have more than 64 bit by iterating the hash function. 3.5.2, one important property which makes DES secure is that the S-boxes are nonlinear. Even though there is a chance you will not generate y U at the output, the likelihood is small.
If you spot any mistakes, please leave a comment in the Disqus box at the bottom of the page.īecause all inputs are 1s and \(IP\), \(PC-1\), \(PC-2\) and the subkey rotations are all simple permutations, we can discount them knowing that they will output all 1s.īecause the subkeys consist of all 1s, we can discount them from the \(f\) function calculation by inverting the S-box input (since \(a \oplus 1 = a^\] Understanding Cryptography SOLUTIONS - Free download as PDF File (.pdf), Text File (.txt) or read online for free. I haven’t yet verified this solution independently. Pairing this frequency table with the list of most common letters in the English language does not yield a key which produces intelligible text. Tallying up the letter frequencies produces the table below: 2.
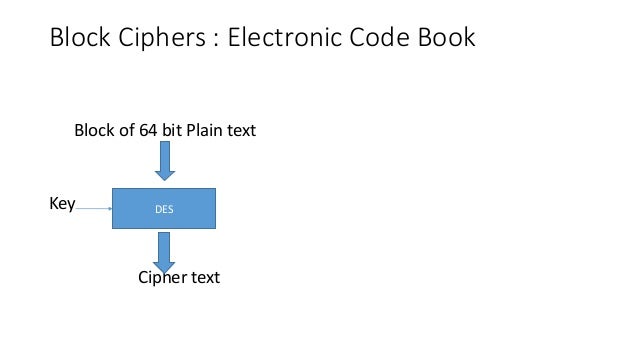
What is the output of the first round of the DES algorithm when the plaintext and the key are both all ones? Solution This solution is verified as correct by the official Solutions for Odd-Numbered Questions manual. Where P plaintext, C ciphertext, E the encryption method, D the decryption method, and k the key. Cryptography understanding-cryptography even-numbered-solutions Understanding cryptography even solutions manual password.


 0 kommentar(er)
0 kommentar(er)
